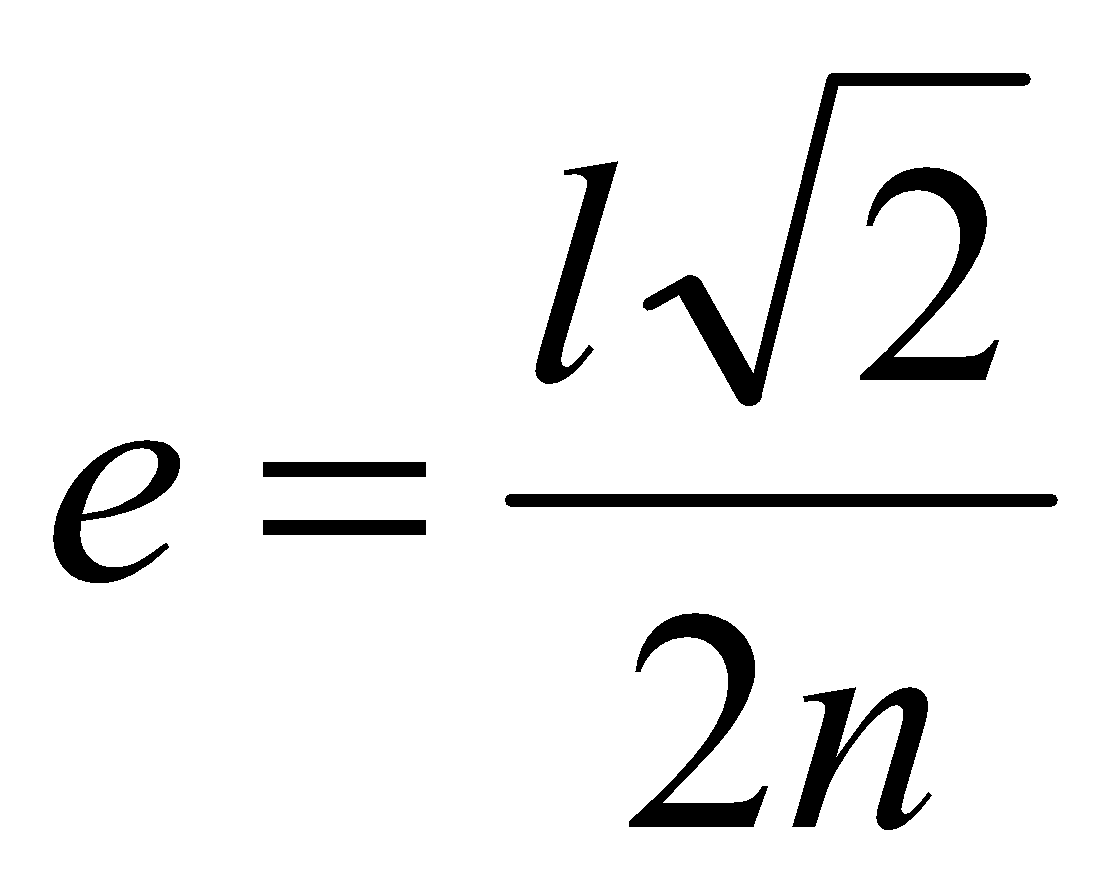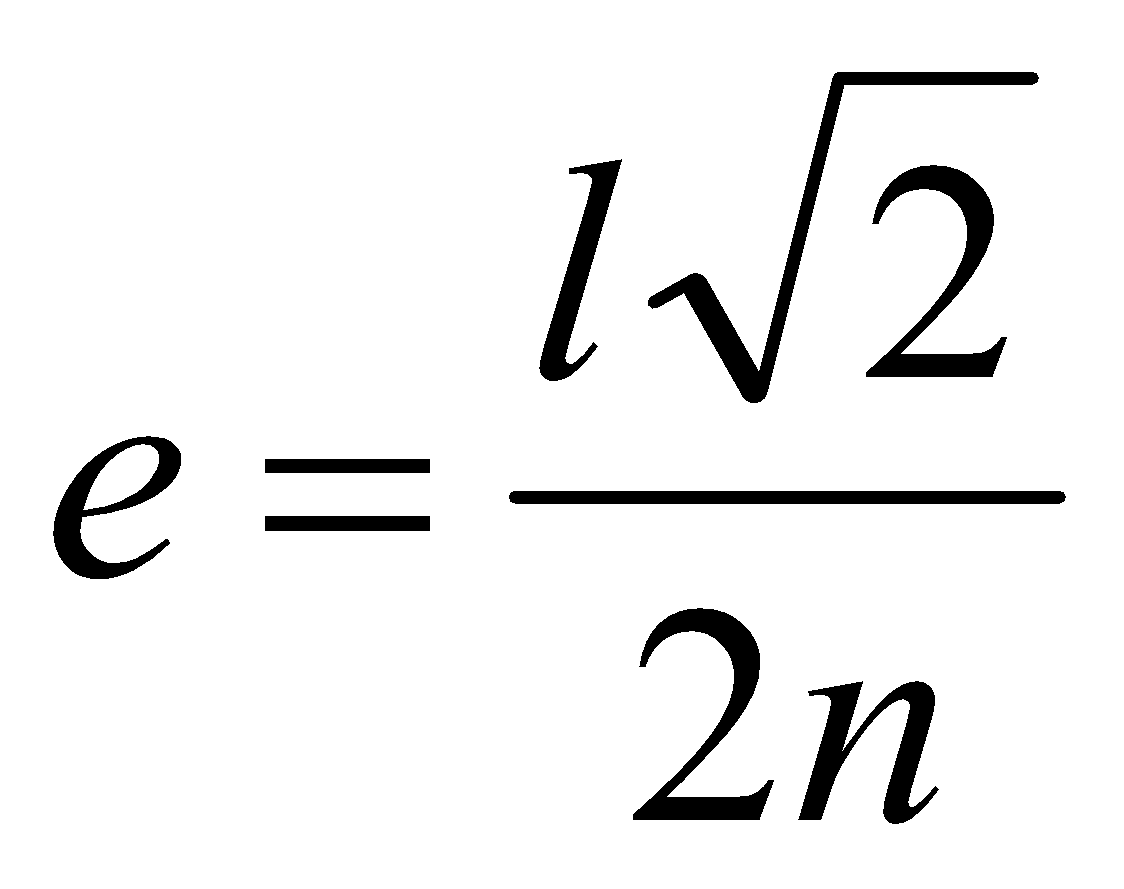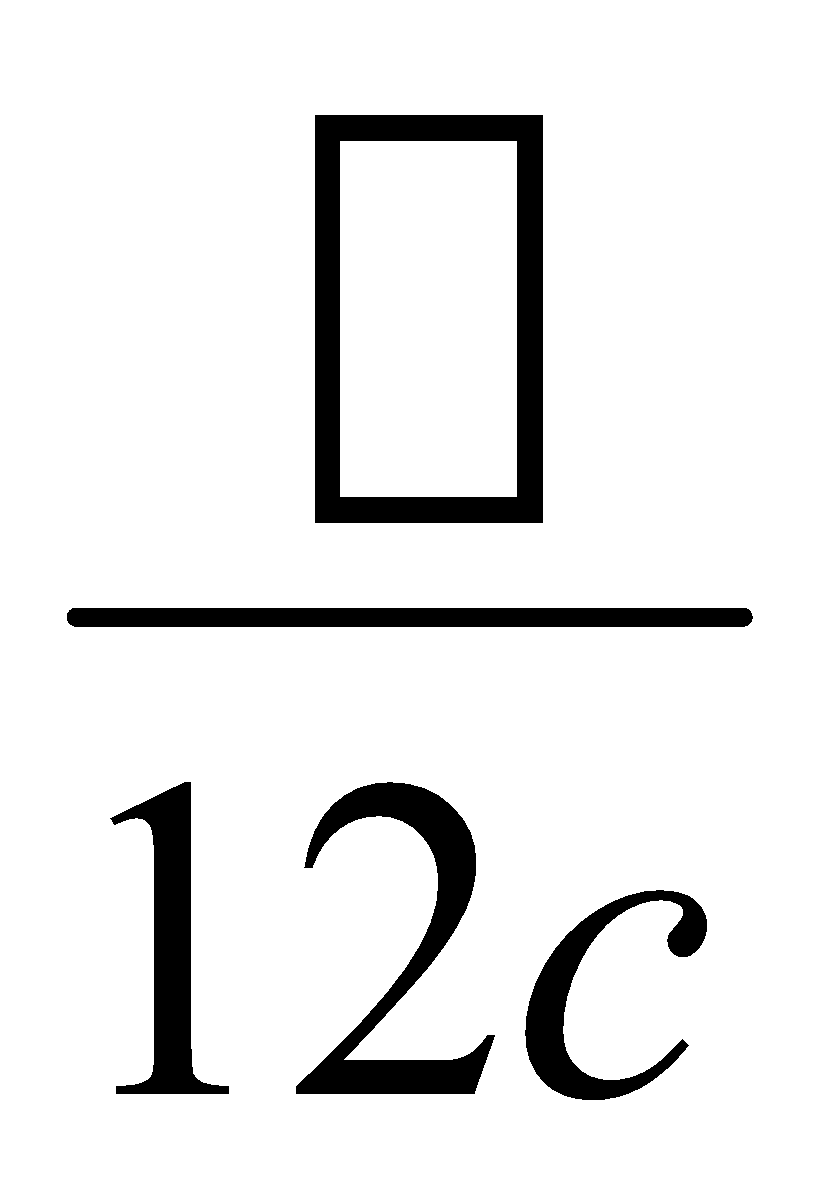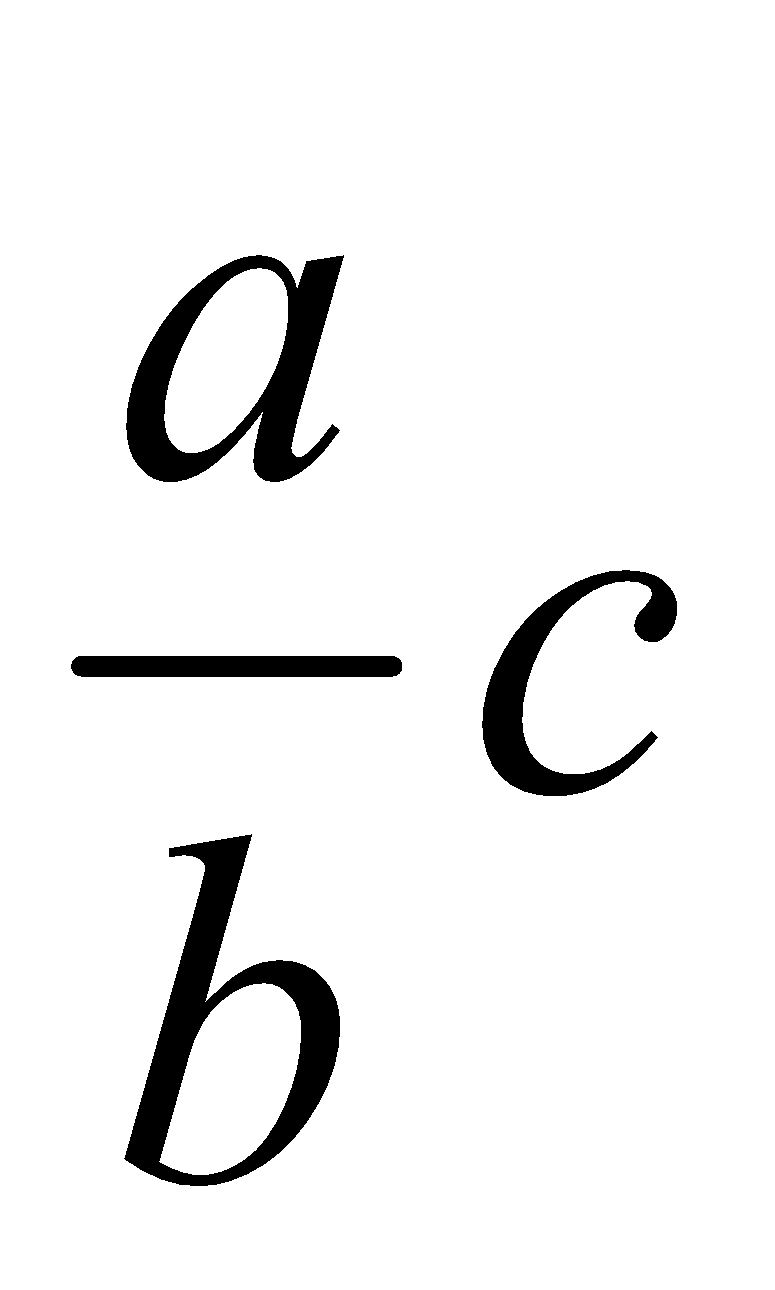Baryons in the IRC Model
Aran David Stubbs, Inframatter Research Center
Abstract
Our model has 3 tiers below leptons and quarks: proto-matter, mezzo-matter, and infra-matter. Each has characteristic tachyons binding together the lower level structures to produce the higher level. Each class of tachyon generates its own granularity constant. The proto-matter is bound by gravitons to form the leptons and quarks. The mezzo-matter is bound by mezzo-tachyons to form the proto-matter. The infra-matter is bound by infra-tachyons to form the mezzo-matter. 2 types of mezzo tachyons bind the mezzo-matter structures: a charge tachyon binding s mezzo-matter (with l=0), and a color tachyon binding structures with l>0. The s structure has 1 infra-tachyon and 1 infra-photon, in 1s orbits. The p structure has 7 of each: among 4 s sub-shells and 1 p. The d structure has 11 s sub-shells, 3 p, and 1 d. Etc. Based on the first 2 leptons, a solution for the energy of the s (charge) structure, and the p (color) structure were deduced, from which the other mezzo structures energies were generated. In the IRC model a trio of proto-quarks can combine in 3 arrangements: a single ring with all 3, which we call negrons; a trio of quarks, the delta class; and a pair forming a diquark bound to an adjacent quark – the Baryons. As the degrees of freedom is large in this situation, the solution space is under specified at present.
Assumptions
- All Tardyons (any object moving slower than the speed of light) are structures, and all structures are tardyons. Structure as used here means anything that can be treated as a unit, but that has constituents.
- The fundamental forces of electro-magnetism, gravity, and the strong force are the result of wave on wave refraction. This slows the wave causing an attraction for the tardyons, and a repulsion for the tachyons.
- Tachyons become trapped in structures when Lv>λ. The Lv in question is the length from the tachyon’s perspective of the orbiting tardyons with Lv ~L0 V/c.
- Centripetal force is 2Ek/r for all types. Where V is small, this reduces to mV2/r.
- The granularity constant h relates the total energy of a photon to its frequency. The standard form E=hν only applies for the photon. The individual pieces follow a more general law E=hc/λP*, where λ is the wavelength, and P* is the number of energy equivalent pieces in a photon (12). This E is the kinetic energy. This form holds for the immediate constituents of each of the elementary particles. Similarly, angular momentum comes in quanta of .
- This granularity constant is generated by the gravitons within the structure.
- Other granularity constants are generated by other tachyons.
- Kepler’s laws are only applicable in a Newtonian framework. Where V is large, a more general form using energy is required. For an electron in isolation near a charge, the stable 1s orbit occurs where r = a0/z, where z is the ratio of the charge on the structure to the charge on a proton, a0 is Bohr’s radius, and r is the distance between the centers of mass of the structure and the electron. The Kinetic Energy of the orbits of the charged structure and the electron total z2E1 where E1 is the energy for z=1. Velocity is derived, not proportionate to z. For many cases z is a net charge, often symbolized as z*.
- The infra-matter luxons in s orbits are synchronized to the gravitons, having 1 orbital cycle in the time a graviton has Þ (a rational >3).
- The electrons have eccentric orbits, aside from the s, with focal length f proportionate to l number. P (l=1) has f of 1s radius /√2 so 2p has eccentricity e of √2/4, 3p has e of √2/6, etc. D (l=2) has twice the focal length of P, so 3d has e of √2/3. Similarly, the proto-matter and infra-matter have orbits with eccentricity of .
Introduction
The standard model has an atom containing a dense nucleus surrounded by clouds of electrons. The nucleus contains discrete neutrons and protons exchanging virtual pions as they travel briskly through a small volume. These nucleons are in turn comprised of trios of quarks also moving briskly, which exchange virtual gluons to cling together.
The IRC model has a nucleus comprised of monoquarks and diquarks in a crystalline structure, surrounded by a photon-like shell comprised of parallel rings. The proton and the neutron are then small nuclei, and aren’t themselves present as such in the larger nuclei. This parallels the salt crystal, where many sodium ions are bound to many chloride ions, not in explicit pairs of sodium chloride.
In our model – the electrons, quarks, diquarks, and the photon-like shell are each structures. These structures contain proto-matter orbiting above pairs of gravitons. The gravitons are low-energy tachyons, travelling much faster than the speed of light. Each kind of proto-matter in turn is a structure. The 2 proto-bosons (the proto-photon and proto-gluon) are comprised of infra-photons above infra-tachyons. All the other required forms of proto-matter are comprised of mezzo-matter above mezzo-tachyons. Each kind of mezzo-matter is comprised of infra-photons above infra-tachyons.
A Sample Baryon
The first baryon discovered, the proton, is a good starting point to look at the family. In the standard model, the proton is comprised of 2 up quarks and a down quark. In the IRC model it is comprised of an up/down diquark, an up quark, and a single ring photon-like shell. The up/down diquark has a proto-up and a proto-down in 2s orbits, with a pair of gravitons in the 1s orbits. Perpendicular to these orbits there is another pair of gravitons in the 1p orbits, topped by a proto-gluon in a 4g orbit. The 4g is the next orbit that has both the eccentricity of the 1p and is a multiple of the number of possible orbits (18 versus 6). The up quark similarly has a pair of gravitons each in the 1s and 1 pair of 1p, a proto-up in a 2s orbit, and a proto-gluon in a 4g orbit.
The kinetic energy of a proto-matter piece or a graviton is proportionate to the 1s orbits energy. A 2s has twice the energy of a 1s, a 3s has thrice, and so forth. The orbits with l>0 are eccentric, and have energy and angular momentum that vary based on where in the orbit the particle is at. A simple equation was generated for the average energy of a luxon (travelling at c), from which a fairly simple equation was generated for the average energy of a tachyon, and an approximation for the average energy of a tardyon. Using these forms an energy equivalent piece count was produced for the various structures. This is denoted as P*. Example: the photon in our model has 2 gravitons and 4 proto-photons, filling 1s, 2s, and 3s sub-shells. P*=1+1+2+2+3+3=12. An exact form was also found for the eccentricity of any orbit in terms of l, n of .
Related to the energy calculation, an angular momentum calculation can be made. For luxons in an n orbit (s or higher), the angular momentum is n small units, where a small units is . The photon has 3 pairs, with equivalent pieces in each, so net L=0. When a tardyon is in an orbit, it is travelling less than c with a velocity that can be easily calculated. If the orbit is n, and it has m extra units of angular momentum, the velocity is . Then b=a+m, and.
The proto-up is the first proto-quark. It contains 4 s sub-shells and 1 p sub-shell of mezzo matter and mezzo tachyons. Each sub-shell is half and half, with a mezzo-tachyon topped by a mezzo-matter structure. There are 2 types of mezzo matter involved, an s and a p. The s mezzo-matter resembles the proto-photon with a single infra photon just above an infra-tachyon in the 1s sub-shell. The p mezzo-matter structure resembles the proto-gluon each of which have 4 s sub-shells and 1 p sub-shells, half and half infra-photons and infra-tachyons.
The proto-down is the second proto-quark. It contains 7 s sub-shells, and 2 p sub-sub-shells. A zeroeth proto-quark would have 2 s sub-shells and no p. The 3rd proto-quark, the proto-strange, has 11s sub-shells, 3 p sub-shells, and 1 d sub-shell. Similarly, the d mezzo-matter has 11s, 3p, and 1d sub-shell. In general, the jth proto-quark has j+1 more s sub-shells than the j-1 quark. All the mezzo matter with l>0 appear to bind the same mezzo tachyon, but we are still calling it the color tachyon.
As the orbits with l>0 are eccentric, only a few arrangements end up as stable, those with a constant angular momentum for the structure. With half of each sub-shell tachyons, the obvious stable situation occurs when all of the tachyons have their angular momentum maximize simultaneously, distributed equally around a circle. For the p sub shell, that has the vectors 120° apart. For the d they are 72° apart, and so forth.
In a few cases a second stable form is possible. When l=3i+1, where i is an integer, a solution with a trio of vectors 60° apart can form. There are then 2i-1 independent trios. For l=1 (i=0), the trio forms a net color, with the central vector reaching its maximum when the 2 outer vectors are at their minimum. The overall sum is twice the average of any of the vectors. Thus red plus anti-blue plus anti-green equal double red. When i>0, these are referred to as “Hues”.
The p sub-shells higher than 1p can also add to the color vector from the 1p sub-shell, but the average intensity doesn’t match, so these are referred to as “Tints”, if they even exist.
The best fit to the observed properties of the proton came with the 3 proto-quarks each in 2s orbits. Other solutions are easily envisioned, and may generate similar but higher energy versions. The 1s energy of the contents of the diquark is about 33.041 MeV, with a proto-up having a rest energy of 17.961 MeV, and the proto-down having a rest energy of 36.534 MeV, the total energy of the diquark is 413.697 MeV. The 1s energy of the contents of the up quark is about 38.967 MeV, giving a total energy for the up quark of 363.653 MeV. The photon-like shell has a single ring with a pair of gravitons in 1s orbits, and a trio of proto-photons in the 2s and a 3s orbit. The 1s energy is 17.880 MeV, giving a total energy of 160.922 MeV for the shell, and 938.272 MeV for the overall proton. This had a slightly small effective diameter of 1.667 fm, compared to a reported 1.681 fm. Gross diameter is 1.839 fm at the charge ring, and a maximum of 1.408 fm perpendicular to the charge ring.
Generalizing
Unfortunately, these calculations were done based on observed energy. To get a generalized solution, energy should be a result rather than an input. A force balance was attempted on the contents of the electron, but ended with 3 unknown probability ratios being treated as 1:1. As the space is under-specified, only cases where at least 1 additional input (often energy) is available can be calculated.
The second known baryon, the neutron, is in our theory an up/down diquark bound to a down quark. A solution with both proto-downs 2s was examined, but the angular momentum was either 2 or 4 small units (depending on whether the angular momentum of the pieces add or subtract), and the reported value is 6 (that is a spin of ½). A solution with a 3s proto-down (in the down) and a 2s proto-down (in the diquark) came out more plausible in the angular momentum. This has a 1s energy in the diquark of 34.345 MeV, for a total diquark energy of 633.941 MeV. The 1s energy in the down quark is 18.095 MeV, for a total down energy of 305.624 MeV, and a neutron energy of 939.565 MeV. Gross diameter is 2.775 fm, effective diameter is 2.476 fm, and maximum diameter perpendicular to the charge rings is 2.570 fm. Note: there isn’t a single ring of proto-photons here, since the overall structure is neutral, but a proto-photon each in the basic structure of the diquark and the down, which explains their much higher energies.
The deltas aren’t baryons in our theory, so the next baryon is the lambda with an up/down diquark and a strange quark. The proto-strange has a rest energy of about 270.722 MeV. Solutions:
- There is a possible solution with a 2s proto-strange, where the 1s has a kinetic energy of 37.7635 MeV, giving a total energy of 794.552 MeV for the Strange. Then the 1s in the diquark is 15.804 MeV, for total diquark energy of 321.131 MeV and total Lambda energy of 1115.683 MeV. The lambda then has a gross diameter of 2.952 fm, an effective diameter of 2.540 fm, and a maximum diameter perpendicular to the charge rings of 2.443 fm. These are well above the overall minima.
- Several possibilities with either a 2s or 3s proto-strange are closer to the minima, but have the wrong L (integer, but not 6).
- The only 3s that is better on any of the measures with the correct L has a 1s energy for the strange quark’s content of 32.752 MeV, giving a total energy to the strange of 757.791 MeV. Then the diquark content has a 1s energy of 17.983 MeV, giving the diquark a total energy of 357.892 MeV. The gross diameter is 2.833 fm, and the effective diameter is 2.491 fm, with a maximum perpendicular diameter of 2.586 fm.
- There are 2 good solutions with the proto-strange in 4s, of which the better has a 1s energy of 23.048 MeV, so a total energy for the strange of 636.518 MeV. Then the diquark has a 1s piece as 25.171 MeV, for a total diquark energy of 479.165 MeV, and total energy for the lambda again 1115.683 MeV. The gross diameter is 2.734 fm, effective diameter of 2.500 fm, and maximum perpendicular diameter of 2.018 fm.
No known baryon requires the Up/Up or Down/Down diquark. These may or may not exist. Some of the heavier transient baryons listed as nucleon family or delta family may include them.
Several other baryons have structures with an up/down diquark, but they are relatively heavy. These include the Charmed Lambda and Bottom Lambda. Looking at some lighter cases, there are several with an up/strange diquark. Like the up/down diquark, this has a charge of 1/3, and can bind to any among up, down, and strange quarks (and maybe heavier as well). Traditionally these are the Sigma +, Sigma 0, and Xi 0 respectively. The down/strange diquark similarly can bind to these 3 monoquarks producing the Sigma 0, Sigma -, and Xi -. Whether the Sigma 0 is US+D or DS+U is unclear.